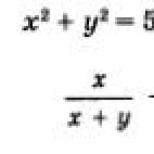The product of algebraic fractions is the square of a binomial. Adding and subtracting algebraic fractions
Abbreviated expression formulas are very often used in practice, so it is advisable to learn them all by heart. Until this moment, it will serve us faithfully, which we recommend printing out and keeping before your eyes at all times:
The first four formulas from the compiled table of abbreviated multiplication formulas allow you to square and cube the sum or difference of two expressions. The fifth is intended for briefly multiplying the difference and the sum of two expressions. And the sixth and seventh formulas are used to multiply the sum of two expressions a and b by their incomplete square of the difference (this is what an expression of the form a 2 −a b+b 2 is called) and the difference of two expressions a and b by the incomplete square of their sum (a 2 + a·b+b 2 ) respectively.
It is worth noting separately that each equality in the table is an identity. This explains why abbreviated multiplication formulas are also called abbreviated multiplication identities.
When solving examples, especially in which the polynomial is factorized, the FSU is often used in the form with the left and right sides swapped:

The last three identities in the table have their own names. The formula a 2 −b 2 =(a−b)·(a+b) is called difference of squares formula, a 3 +b 3 =(a+b)·(a 2 −a·b+b 2) - sum of cubes formula, A a 3 −b 3 =(a−b)·(a 2 +a·b+b 2) - difference of cubes formula. Please note that we did not name the corresponding formulas with rearranged parts from the previous table.
Additional formulas
It wouldn’t hurt to add a few more identities to the table of abbreviated multiplication formulas.
Areas of application of abbreviated multiplication formulas (FSU) and examples
The main purpose of abbreviated multiplication formulas (fsu) is explained by their name, that is, it consists in briefly multiplying expressions. However, the scope of application of FSU is much wider, and is not limited to short multiplication. Let's list the main directions.
Undoubtedly, the central application of the abbreviated multiplication formula was found in performing identical transformations of expressions. Most often these formulas are used in the process simplifying expressions.
Example.
Simplify the expression 9·y−(1+3·y) 2 .
Solution.
In this expression, squaring can be performed abbreviated, we have 9 y−(1+3 y) 2 =9 y−(1 2 +2 1 3 y+(3 y) 2). All that remains is to open the brackets and bring similar terms: 9 y−(1 2 +2 1 3 y+(3 y) 2)= 9·y−1−6·y−9·y 2 =3·y−1−9·y 2.
Frankly, these are formulas that any seventh grade student should remember. It is simply impossible to study algebra even at school level and not know the formula for the difference of squares or, say, the square of a sum. They appear all the time when simplifying algebraic expressions, reducing fractions, and can even help with arithmetic calculations. Well, for example, you need to calculate in your head: 3.16 2 - 2 3.16 1.16 + 1.16 2. If you start calculating it head-on, it will turn out long and boring, but if you use the squared difference formula, you will get the answer in 2 seconds!
So, seven formulas of “school” algebra that everyone should know:
| Name | Formula |
| Square of the sum | (A + B) 2 = A 2 + 2AB + B 2 |
| Squared difference | (A - B) 2 = A 2 - 2AB + B 2 |
| Difference of squares | (A - B)(A + B) = A 2 - B 2 |
| Cube of sum | (A + B) 3 = A 3 + 3A 2 B + 3AB 2 + B 3 |
| Difference cube | (A - B) 3 = A 3 - 3A 2 B + 3AB 2 - B 3 |
| Sum of cubes | A 3 + B 3 = (A + B)(A 2 - AB + B 2) |
| Difference of cubes | A 3 - B 3 = (A - B)(A 2 + AB + B 2) |
Please note: there is no formula for the sum of squares! Don't let your imagination go too far.
What is the easiest way to remember all these formulas? Well, let's say, see certain analogies. For example, the formula for the squared sum is similar to the formula for the squared difference (the difference is only in one sign), and the formula for the cube of the sum is similar to the formula for the cube of the difference. Further, in the formulas for the difference of cubes and the sum of cubes, we see something similar to the square of the sum and the square of the difference (only coefficient 2 is missing).
But these formulas (like any others!) are best remembered in practice. Solve more examples of simplifying algebraic expressions, and all the formulas will be remembered by themselves.
Curious students will probably be interested in summarizing the facts presented. For example, there are formulas for the square and cube of a sum. What if we consider expressions like (A + B) 4, (A + B) 5 and even (A + B) n, where n is an arbitrary natural number? Is it possible to see any pattern here?
Yes, such a pattern exists. An expression of the form (A + B) n is called Newton's binomial. I recommend that inquisitive schoolchildren deduce the formulas for (A + B) 4 and (A + B) 5 themselves, and then try to see the general law: compare, for example, the degree of the corresponding binomial and the degree of each of the terms that are obtained by opening the brackets; compare the degree of a binomial with the number of terms; try to find patterns in the coefficients. We will not delve into this topic now (this requires a separate conversation!), but will only write down the finished result:
(A + B) n = A n + C n 1 A n-1 B + C n 2 A n-2 B 2 + ... + C n k A n-k B k + ... + B n .
Here C n k = n!/(k! (n-k)!).
I remind you that n! - this is 1 2 ... n - the product of all natural numbers from 1 to n. This expression is called factorial of n. For example, 4! = 1 2 3 4 = 24. The factorial of zero is considered equal to one!
What can be said about the difference of squares, difference of cubes, etc.? Is there any pattern here? Is it possible to give a general formula for A n - B n ?
Yes, you can. Here is the formula:
A n - B n = (A - B)(A n-1 + A n-2 B + A n-3 B 2 + ... + B n-1).
Moreover, for odd degrees n there is a similar formula for the sum:
A n + B n = (A + B)(A n-1 - A n-2 B + A n-3 B 2 - ... + B n-1).
We will not derive these formulas now (by the way, it is not very difficult), but knowing about their existence is certainly useful.
Ordinary fractions.
Adding algebraic fractions
Remember!
You can only add fractions with the same denominators!
You can't add fractions without conversions

You can add fractions

When adding algebraic fractions with like denominators:
- the numerator of the first fraction is added to the numerator of the second fraction;
- the denominator remains the same.
Let's look at an example of adding algebraic fractions.

Since the denominator of both fractions is “2a”, it means that the fractions can be added.
Let's add the numerator of the first fraction with the numerator of the second fraction, and leave the denominator the same. When adding fractions in the resulting numerator, we present similar ones.

Subtracting algebraic fractions
When subtracting algebraic fractions with like denominators:
- The numerator of the second fraction is subtracted from the numerator of the first fraction.
- the denominator remains the same.
Important!
Be sure to include the entire numerator of the fraction you are subtracting in parentheses.
Otherwise, you will make a mistake in the signs when opening the brackets of the fraction you are subtracting.
Let's look at an example of subtracting algebraic fractions.
Since both algebraic fractions have a denominator of “2c”, this means that these fractions can be subtracted.
Subtract the numerator of the second fraction “(a − b)” from the numerator of the first fraction “(a + d)”. Don't forget to put the numerator of the fraction you are subtracting in parentheses. When opening parentheses, we use the rule for opening parentheses.

Reducing algebraic fractions to a common denominator
Let's look at another example. You need to add algebraic fractions.

Fractions cannot be added in this form because they have different denominators.
Before adding algebraic fractions, they must be bring to a common denominator.
The rules for reducing algebraic fractions to a common denominator are very similar to the rules for reducing ordinary fractions to a common denominator. .
As a result, we should get a polynomial that will be divided without a remainder into each of the previous denominators of the fractions.
To reduce algebraic fractions to a common denominator you need to do the following.
- We work with numerical coefficients. We determine the LCM (least common multiple) for all numerical coefficients.
- We work with polynomials. We define all the different polynomials in the greatest powers.
- The product of the numerical coefficient and all various polynomials in the greatest powers will be the common denominator.
- Determine what you need to multiply each algebraic fraction by to get a common denominator.
Let's return to our example.

Consider the denominators “15a” and “3” of both fractions and find a common denominator for them.
- We work with numerical coefficients. Find the LCM (the least common multiple is a number that is divisible by each numerical coefficient without a remainder). For "15" and "3" it is "15".
- We work with polynomials. It is necessary to list all polynomials in the greatest powers. In the denominators "15a" and "5" there are only
one monomial - “a”. - Let’s multiply the LCM from step 1 “15” and the monomial “a” from step 2. We get “15a”. This will be the common denominator.
- For each fraction, we ask ourselves the question: “What should we multiply the denominator of this fraction by to get “15a”?”
Let's look at the first fraction. This fraction already has a denominator of “15a,” which means it doesn’t need to be multiplied by anything.
Let's look at the second fraction. Let’s ask the question: “What do you need to multiply “3” by to get “15a”?” The answer is “5a”.
When reducing a fraction to a common denominator, multiply by “5a” both numerator and denominator.

A shortened notation for reducing an algebraic fraction to a common denominator can be written using “houses”.
To do this, keep the common denominator in mind. Above each fraction at the top “in the house” we write what we multiply each of the fractions by.

Now that the fractions have the same denominators, the fractions can be added.
Let's look at an example of subtracting fractions with different denominators.

Consider the denominators “(x − y)” and “(x + y)” of both fractions and find the common denominator for them.
We have two different polynomials in the denominators "(x − y)" and "(x + y)". Their product will be the common denominator, i.e. “(x − y)(x + y)” is the common denominator. 

Adding and subtracting algebraic fractions using abbreviated multiplication formulas
In some examples, abbreviated multiplication formulas must be used to reduce algebraic fractions to a common denominator.
Let's look at an example of adding algebraic fractions, where we will need to use the difference of squares formula.

In the first algebraic fraction the denominator is “(p 2 − 36)”. Obviously, the difference of squares formula can be applied to it.

After decomposing the polynomial “(p 2 − 36)” into the product of polynomials
“(p + 6)(p − 6)” it is clear that the polynomial “(p + 6)” is repeated in fractions. This means that the common denominator of the fractions will be the product of polynomials “(p + 6)(p − 6)”.
In this article we will look at basic operations with algebraic fractions:
- reducing fractions
- multiplying fractions
- dividing fractions
Let's start with reduction of algebraic fractions.
It would seem that, algorithm obvious.
To reduce algebraic fractions, need to
1. Factor the numerator and denominator of the fraction.
2. Reduce equal factors.
However, schoolchildren often make the mistake of “reducing” not the factors, but the terms. For example, there are amateurs who “reduce” fractions by and get as a result , which, of course, is not true.
Let's look at examples:
1.
Reduce fraction: 
1. Let us factorize the numerator using the formula of the square of the sum, and the denominator using the formula of the difference of squares


2. Divide the numerator and denominator by

2.
Reduce fraction: 
1. Let's factorize the numerator. Since the numerator contains four terms, we use grouping.
2. Let's factorize the denominator. We can also use grouping.
3. Let's write down the fraction that we got and reduce the same factors:

Multiplying algebraic fractions.
When multiplying algebraic fractions, we multiply the numerator by the numerator, and multiply the denominator by the denominator.
 Important! There is no need to rush to multiply the numerator and denominator of a fraction. After we have written down the product of the numerators of the fractions in the numerator, and the product of the denominators in the denominator, we need to factor each factor and reduce the fraction.
Important! There is no need to rush to multiply the numerator and denominator of a fraction. After we have written down the product of the numerators of the fractions in the numerator, and the product of the denominators in the denominator, we need to factor each factor and reduce the fraction.
Let's look at examples:
3. Simplify the expression:

1. Let’s write the product of fractions: in the numerator the product of the numerators, and in the denominator the product of the denominators:


2. Let's factorize each bracket:

Now we need to reduce the same factors. Note that the expressions and differ only in sign: ![]() and as a result of dividing the first expression by the second we get -1.
and as a result of dividing the first expression by the second we get -1.

So, 
We divide algebraic fractions according to the following rule:
 That is To divide by a fraction, you need to multiply by the "inverted" one.
That is To divide by a fraction, you need to multiply by the "inverted" one.
We see that dividing fractions comes down to multiplying, and multiplication ultimately comes down to reducing fractions.
Let's look at an example:
4. Simplify the expression:

This lesson will cover adding and subtracting algebraic fractions with like denominators. We already know how to add and subtract common fractions with like denominators. It turns out that algebraic fractions follow the same rules. Learning to work with fractions with like denominators is one of the cornerstones of learning how to work with algebraic fractions. In particular, understanding this topic will make it easy to master a more complex topic - adding and subtracting fractions with different denominators. As part of the lesson, we will study the rules for adding and subtracting algebraic fractions with like denominators, and also analyze a number of typical examples
Rule for adding and subtracting algebraic fractions with like denominators
Sfor-mu-li-ru-em pra-vi-lo slo-zhe-niya (you-chi-ta-niya) al-geb-ra-i-che-skih fractions from one-on-to-you -mi know-me-na-te-la-mi (it coincides with the analogous rule for ordinary shot-beats): That is for addition or calculation of al-geb-ra-i-che-skih fractions with one-to-you know-me-on-the-la-mi necessary -ho-di-mo-compile a corresponding al-geb-ra-i-che-sum of numbers, and the sign-me-na-tel leave without any.
We understand this rule both for the example of ordinary ven-draws and for the example of al-geb-ra-i-che-draws. hit.
Examples of applying the rule for ordinary fractions
Example 1. Add fractions: .
Solution
Let's add the number of fractions, and leave the sign the same. After this, we decompose the number and sign into simple multiplicities and combinations. Let's get it: ![]() .
.
Note: a standard error that is allowed when solving similar types of examples, for -klu-cha-et-sya in the following possible solution: ![]() . This is a gross mistake, since the sign remains the same as it was in the original fractions.
. This is a gross mistake, since the sign remains the same as it was in the original fractions.
Example 2. Add fractions: .
Solution
This one is in no way different from the previous one: .
Examples of applying the rule for algebraic fractions
From ordinary dro-beats, we move to al-geb-ra-i-che-skim.
Example 3. Add fractions: .
Solution: as already mentioned above, the composition of al-geb-ra-i-che-fractions is in no way different from the word the same as usual shot-fights. Therefore, the solution method is the same: .
Example 4. You are the fraction: .
Solution
You-chi-ta-nie of al-geb-ra-i-che-skih fractions from addition only by the fact that in the number pi-sy-va-et-sya difference in the number of used fractions. That's why .
Example 5. You are the fraction: .
Solution: .
Example 6. Simplify: .
Solution: .
Examples of applying the rule followed by reduction
In a fraction that has the same meaning in the result of compounding or calculating, combinations are possible nia. In addition, you should not forget about the ODZ of al-geb-ra-i-che-skih fractions.
Example 7. Simplify: .
Solution: .
Wherein . In general, if the ODZ of the initial fractions coincides with the ODZ of the total, then it can be omitted (after all, the fraction is being in the answer, will also not exist with the corresponding significant changes). But if the ODZ of the used fractions and the answer doesn’t match, then the ODZ needs to be indicated.
Example 8. Simplify: .
Solution: . At the same time, y (the ODZ of the initial fractions does not coincide with the ODZ of the result).
Adding and subtracting fractions with different denominators
To add and read al-geb-ra-i-che-fractions with different know-me-on-the-la-mi, we do ana-lo -giyu with ordinary-ven-ny fractions and transfer it to al-geb-ra-i-che-fractions.
Let's look at the simplest example for ordinary fractions.
Example 1. Add fractions: .
Solution:
Let's remember the rules for adding fractions. To begin with a fraction, it is necessary to bring it to a common sign. In the role of a general sign for ordinary fractions, you act least common multiple(NOK) initial signs.
Definition
The smallest number, which is divided at the same time into numbers and.
To find the NOC, you need to break down the knowledge into simple sets, and then select everything there are many, which are included in the division of both signs.
; . Then the LCM of numbers must include two twos and two threes: .
After finding the general knowledge, it is necessary for each of the fractions to find a complete multiplicity resident (in fact, in fact, to pour the common sign onto the sign of the corresponding fraction).
Then each fraction is multiplied by a half-full factor. Let's get some fractions from the same ones you know, add them up and read them out. -studied in previous lessons.
Let's eat: ![]() .
.
Answer:.
Let's now look at the composition of al-geb-ra-i-che-fractions with different signs. Now let’s look at the fractions and see if there are any numbers.
Adding and subtracting algebraic fractions with different denominators
Example 2. Add fractions: .
Solution:
Al-go-rhythm of the decision ab-so-lyut-but ana-lo-gi-chen to the previous example. It’s easy to take the common sign of the given fractions: and additional multipliers for each of them.
![]() .
.
Answer:.
So, let's form al-go-rhythm of addition and calculation of al-geb-ra-i-che-skih fractions with different signs:
1. Find the smallest common sign of the fraction.
2. Find additional multipliers for each of the fractions (indeed, the common sign of the sign is given -th fraction).
3. Up-to-many numbers on the corresponding up-to-full multiplicities.
4. Add or calculate fractions, using the right-of-minor additions and calculating fractions with the same knowledge -me-na-te-la-mi.
Now let's look at an example with fractions, in the sign of which there are letters you -nia.